Resistance networks
1. The cube
Twelve resistors in the form
of a cube. The problem is to find the resistance (R) between the points A and B on each
cube. Each arm of the cube has a resistance r.
There are three possible connections.
(a) We can take any path through the network between the
points A and B. Such a path could be ACDB.
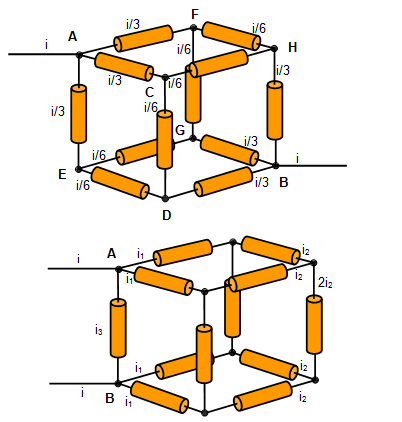
IR = i/3 r + i/6 r + i/3 r = I 5/6 r
Therefore:
Total
resistance (R) = 5/6 r
(b) By
symmetry
i
1 = 5i
2i
3 = 14 i
2i = 24
i
2Therefore:
Total resistance (R) = 7/12
r

(c)
By symmetry
Potential at C = potential at D
Potential
at E = potential at F
Potential at C = potential at D = potential at E = potential at
F
Therefore there is no current in CE or DF
The resistance of the faces ACBD
and GEHF each have a resistance r
The resistance from A to B along the path A to face
GEHF to B is 3r
Therefore resistance from A to B (R) is given by:
1/R = 1/r
+ 1/3r = [4/3]r R = [3/4]r
2. The infinite chain
The problem here is to find the
resistance (R) due to the infinite chain of resistors each of resistance r connected as
shown.


Since the chain is infinite we can think of it as being
equivalent to the following circuit:
Therefore:
R = 2r
+ rR/[r + R] = 2.732r
A VERSION IN WORD IS AVAILABLE ON THE SCHOOLPHYSICS CD





